Glossaire

Payne Effect

The Payne effect is the decrease in the complex modulus of a filled, crossed-linked elastomer system with increasing deformation amplitude.  

If the sample is deformed sinusoidally, the strain follows the ContrainteLa Contrainte est définie par un niveau de force appliquée sur un échantillon d’une section bien définie. (Contrainte = force/surface). Les échantillons qui possèdent une section rectangulaire ou circulaire peuvent être comprimés ou étirés. Les matériaux élastiques comme les élastomères peuvent être étirés jusqu’à 5 à 10 fois leur longueur initiale.stress with a time delay. The decrease in the shear modulus shows a non-linear behavior and can essentially be attributed to the so-called filler-filler interaction (see Figure 1). Different effects contribute to the overall strength of the elastomer:

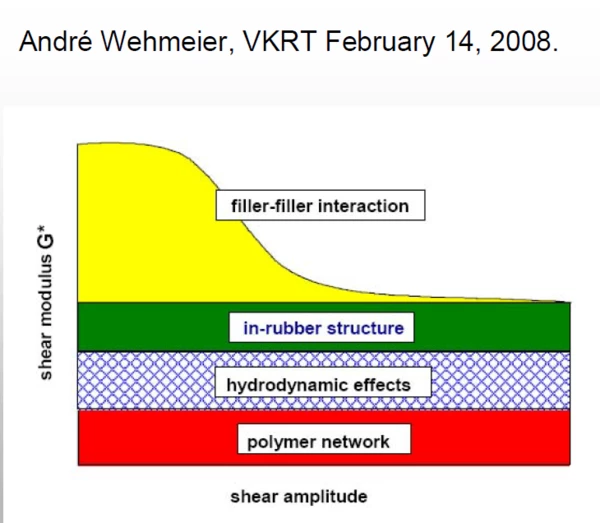
Modulus of the unfilled rubber network

The contribution of the rubber matrix of the (unfilled) rubber network to the total stiffness is independent of the amplitude.

Hydrodynamic effect of filler particles

Hydrodynamic effect of the filler particles (e.g. due to large carbon black particles N8 … N9):

The inelastic filler particles do not participate in elongation and cause higher intrinsic polymer strain compared to the applied macroscopic strain. This effect depends on the volume filling level, but is independent of the load amplitude and is a significant contribution to the overall stiffness.

In-rubber structures

There are filler/matrix interactions (e.g. due to small to medium filler clusters with  N1…, N2..., to N5…)

Filler-matrix interactions:
Parts of the rubber are immobilized in the filler structure. This immobilization also contributes to the overall stiffness independent of the amplitude.

Filler-filler Interactions

The filler-filler interaction is essentially responsible for the decrease in the modulus. Due to the mechanical load, the carbon black agglomerates (clusters) are broken, resulting in a decreasing stiffness. Active carbon black or silica can form a filler-filler network within the polymer matrix, offering high resistance to the small amplitudes. If the amplitude gets too large, this network breaks down and as a result, the G* modulus decreases drastically. For large deformations, the contribution of the filler-filler network to the complex modulus virtually disappears.

The decrease in the modulus is non-linear. This non-linearity is due to hysteresis losses, occurring during degradation of the filler network as well as the release of the polymer trapped in the filler network, which can then contribute to the elongation again.