Introduction
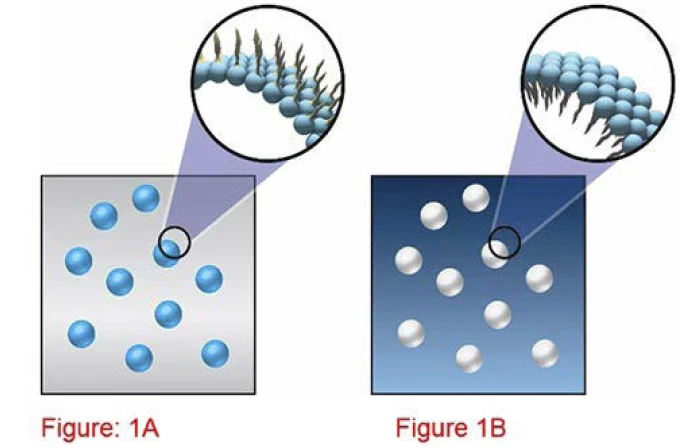
An emulsion is a system with a liquid continuous phase and a dispersed phase of liquid droplets. The two types of emulsions which are most common are oil-in-water emulsions and water-in-oil emulsion (Figure 1). In an oil-in-water emulsion, the continuous phase is water and the dispersed phase is oil, while in a water-in-oil emulsion, the continuous phase is oil, while the dispersed phase is water.
Whether a water-in-oil emulsion turns (or inverts) into an oil-in-water emulsion depends upon the volume fraction of both phases and the emulsifier. An emulsifier is a material that stabilizes an emulsion by adsorbing at the oil water interface. Surfactants are the most common form of emulsifiers.
Emulsion rheology tends to have a very strong dependence on the volume fraction of the dispersed phase as well as the droplet size. The rheological parameters of key interest are the viscosity, normal stress, visco-elasticity and the Yield StressYield stress is defined as the stress below which no flow occurs; literally behaves like a weak solid at rest and a liquid when yielded.yield stress. Low- to medium-concentration emulsions do not tend to exhibit any Yield StressYield stress is defined as the stress below which no flow occurs; literally behaves like a weak solid at rest and a liquid when yielded.yield stress.
On increasing the volume fraction of droplets, a point of phase inversion is reached. However, if the emulsion droplets are stabilized by surfactant or particles, the droplets can remain stable even as the volume fraction approaches 1. Dense or concentrated emulsions tend to exhibit interesting rheological properties such as Yield StressYield stress is defined as the stress below which no flow occurs; literally behaves like a weak solid at rest and a liquid when yielded.yield stress and high viscoelasticity as volume fraction of the dispersed phase exceeds that of close packed spheres configuration (Φ = 0.74 for monodisperse deformable systems). According to Princen and Kriss [1], the Yield StressYield stress is defined as the stress below which no flow occurs; literally behaves like a weak solid at rest and a liquid when yielded.yield stress (σy) generated in such dense emulsions depends upon the volume fraction of the droplets and is given by:
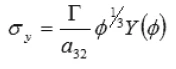
Where Y(Φ) is an empirical function given by;
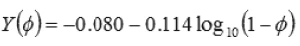
Here, Φ is the volume fraction of the droplets, Γ is the interfacial tension and a32 is the volume to surface drop radius.
To make practical use of this theory, it is necessary to measure the Yield StressYield stress is defined as the stress below which no flow occurs; literally behaves like a weak solid at rest and a liquid when yielded.yield stress of an emulsion at a number of user defined volume fractions (concentrations). If the user has knowledge of the interfacial tension and droplet radius, the data can then be analyzed to see the applicability of the Princen and Kriss model for a specific emulsion sample.
Droplets having radii of about 1 microns or smaller are strongly influenced by Brownian motion and show liquid like behavior at low frequencies and cannot be described using the above analysis.
Experimental
- This experimental test exists as a pre-configured sequence in the rSpace software which is designed to run on a Kinexus rotational rheometer1.
- The sequence determines yield stress by means of a stress ramp at a range of user defined volume fractions and displays a plot of yield stress against concentration which can be exported for further analysis.
- This test is only applicable to samples with high volume fractions, even though the analysis will report a yield stress for all samples tested, hence user disgression is required.
1Please note that a parallel plate geometry or a cylindrical geometry can also be used. A sand blasted geometry should be considered if the material is likely to show wall slip effects. Larger geometries are useful for measurements at low torques, which are more likely to be encountered at lower frequencies. The use of a solvent trap is also recommended for these tests since evaporation of solvent (e.g., water) around the edges of the measuring system can invalidate the test, particularly when working at higher temperatures.